WMAP 2009 Temperature -215 to 206 Picture 1

Planck Scale 1 to 1 Temperature -514 to 517 Picture 2

WMAP 2009 Temperature -500 to 500 Picture 3

Planck Scale 1 to 1 Temperature -500 to 500 Picture 4

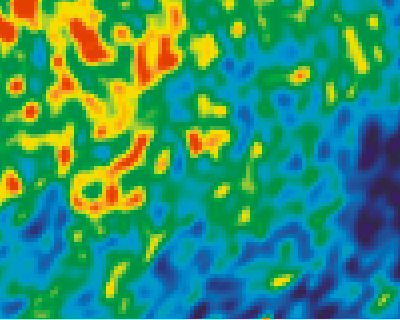
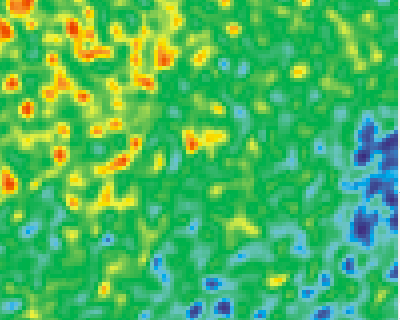
All the images are from the same area in the sky. The enlargement is 400 %
Picture 1 and Picture 2 are the raw pictures. The colour schemes of both pictures is different and are explained below.
Picture 3 and Picture 4 are the modified pictures. The colour scheme of both pictures is the same.

        0,0  ---------- 1024,0                860,240 -------- 960,240
         |                 |                    |               |
         |                 |                    |               |
         |                 |                    |               |
       512,0 ---------- 1024,512              860,320-------- 960,320  
         
            Source Picture                      400 % enlargement
                                 Figure 1

Figure 1 shows the position of the pictures. The top left corners has the coordinates 860,240. The bottom right corner has the coordinates 960,320.

The highest value of the Planck Picture 2 (and Picture 4) is at coordinate 879,247. This point has a temperature of 517 micro Kelvin. This is the brown point near the top left corner

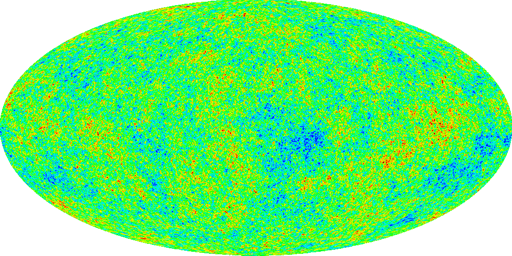
For a copy of the WMAP 2009 source file go to: Ilc_9yr_moll4096.png
For a copy of the Planck source file go to: 735683main_pia16873-full_full.jpg

Picture comparison

When you compare Picture 3 with Picture 4 the difference is striking.

• First in Picture 3 the temperature range is between -200 micro Kelvin and plus 200 micro Kelvin. In Picture 4 the temperature range is between -500 and plus 500.
• The temparture difference between pixels in Picture 4 is much larger than in Picture 3. In Picture 3 the temperature of many adjacent pixels is almost the same. In Picture 4 this is not the case.

Filters

Plank Scale 3 to 1 Temperature -490 to 431 Picture 3

Planck Scale 5 to 1 Temperature -446 to 323 Picture 4

• The left picture shows the Plank data reprocessed using a filter of 3 to 1. When you compare this image with the WMAP 2009 data the similarity is hugh.
• The right picture shows the Plank data reprocessed using a filter of 5 to 1. The left picture is better.

CMBR pictures

Planck Scale 1 to 1 Temperature -514 to 517 Picture 5

Planck Scale 5 to 1 Temperature -446 to 323 Picture 6

Planck Scale 25 to 1 Temperature -307 to 163 Picture 7

• The top picture shows the original planck data.
• The middle picture uses a filter scheme of 5 to 1. This picture represents the WMAP data
• The bottom picture uses a filter scheme of 25 to 1. This picture represents the COBE data.

Colour Investigation

The biggest problem is to convert source colours to temperatures to destination colours. In order to calculate the temperature of the planck 2013 data you have to visit: http://planck.caltech.edu/pub/2013results/Planck_2013_results_01.pdf the top of page 25 ( Planck Collaboration: The Planck mission )
The temperature range goes from -500 to 500. There are 5 regions

Red 0 0 255 255 255 103 Green 9 221 237 180 75 0 Blue 255 255 217 0 0 0 colour blue white red brown temperature -500 -177 -6 161 329 500 region 1 2 3 4 5 Planck Source Colour Scheme Figure 2

The colour of the Planck picture consists of 4 regions and 5 colours: blue(cold), cyan, green, yellow and red(warm)

• The top line represents the value of the colour red. The colour red is only used for the warm temperatures.
• The middle line represents the value of the colour green.
• The bottom line represents the value of the colour blue. The colour blue is used only for the cold temperatures.

The destination colours of the Planck pictures follow a lineair scheme.

Red 0 128 255 255 255 128 Green 0 128 255 255 255 255 255 128 0 0 Blue 128 255 255 255 128 0 0 colour blue cyan green yellow red temperature -500 -400 -300 -200 -100 0 100 200 300 400 500 region 1 2 3 4 Planck/WMAP Destination Colour Scheme Figure 3

The colour of the Planck picture consists of 4 regions and 5 colours: blue(cold), cyan, light, yellow and red(warm)
The scheme is only an approximation
The Planck Source scheme use for each temperature 3 colours. The Planck destination scheme 2.

• The top line represents the value of the colour red. The colour red is mainly used for the warm temperatures but also for the cold temperatures.
• The middle line represents the value of the colour green.
• The bottom line represents the value of the colour blue. The colour blue is mainly used for the cold temperatures but also for the hot temperatures.

The WMAP 2009 colour scheme resembles the Planck destination scheme but also uses for each temperature 3 colours. At the blue side it also uses the colour black.

In order to calculate the temperature of the planck 2013 data you have to visit: http://arxiv.org/abs/1212.5225 the top of page 89 ( 9 Year WMAP Observations: Final Maps and Results )
The temperature range goes from -200 to 200. There are 9 regions

Red 33 20 2 8 30 32 3 142 236 226 182 Green 14 63 130 150 162 161 145 185 221 142 26 Blue 96 132 196 203 183 168 72 12 5 33 26 colour blue temperature -200 -115 -35 -18 9 18 61 114 143 178 200 region 1 2 3 4 5 6 7 8 9 WMAP 2009 Source Colour Scheme Figure 4

The most vivid explanation of the CMB Power Spectrum is by Edward L. Whight in the document: Cosmic Microwave Background Anisotropy .
Here you can read: "The angular power spectrum APS of the anisotropy of the CMB contains information about the Universe. This APS is a plot of how much the temperature varies from point to point. Ell=10 means that there are ten cycles in the fluctuation around the whole sky, while ell=100 means that there are 100 cycles around the sky.
That means that the CMB radiation sky map is a superposition from all the individual sky maps from l = 2 to l = 1000. The question is what is involved to calculate the sky map for each individual l value.
That this calculation is very difficult becomes clear

• if you study the sky map for l = 2. In that case there are two hot and two cold spots. The position of the first hot spot is almost free (left side). The intensity I2 is a free parameter.
• if you study the sky map for l = 4 there are 4 hot and cold spots. The position of the first hot spot covers an area 50% of the case of l = 2. The intensity I4 again is a free parameter.
• In general for each invidual l value you have to calculate the optimum position of two hot spots and the intensity parameter called Il. The sum of all these calculated sky maps should be the observed sky map.

A simpler case is to study not the whole sky but a circle. In that case the CMB radiation is a superposition/summation for l = 1 to 100 for alpha going from 0 to 360 of the function:

R(l)*sin(l * alpha * pi/180 + beta(l)).

These are "open" harmonic waves. See also Harmonic The circles studied follow a meridian, that means they go through the North and South pole.
The following documents show this data in increments of 0.25 degrees:

WMAP database 10.htm , Planck database 10.htm , WMAP database 45.htm , Planck database 45.htm , WMAP database 90.htm , Planck database 90.htm

The layout for each html file is the following:

(Html) (PRE) WMAP database 45.htm 0 198,039215686275 1039 73,8546457699606 (/PRE) (/HTML)

Figure 5 Instead of ( read < . Instead of ) read <

• The first three lines are text. The function as a header
• The lines 4 to 1043 contain data. The first value is an index. The second value is the temperature
• The last two lines are text. The function as a trailer.

WMAP 45 Source Picture 8

Picture 8 shows the 1440 values of the file : "WMAP database 45.htm". The value 45 is the hour angle in degrees.

WMAP 45 Simulation Picture 9

Figure 9 shows the simulated curve of the file : "WMAP database 45.htm". The number of l values calculated is 61. In that case 61 R values and 61 Beta values have to be calculated.

1. The program starts with initial R values of 0 and intial Beta values of 0. This are also called the old R and Beta values.
2. Next delta_R and delta_Beta are calculated.
   delta_R values are random numbers between -0.5 and +0.5. delta_Beta values are random numbers between -0.25 and +0.25.
3. New R values are calculated using R new = R old + delta_R. The same with new Beta values.
4. Using R(l)*sin(l * alpha * pi/180 + beta(l)) and with alpha going from 0 to 1439 a new WMAP function is calculated.
5. At the same time an Error is calculated, with is the difference between the value in the source file (for that angle) and the calculated WMAP value. That Error value is squared. And a total Error value is updated.
6. When all the 1440 WMAP values are calculated the new_Total_Error value is compared with the old_Total_Error Value. When the new value is larger nothing happens. When the new value is smaller the old R values are replaced with the new R values. The same for the old Beta values.

Figure 6 WMAP 45 R and Beta

Figure 6 show the R and Beta values of 4 different simulations of the file : "WMAP database 45.htm" for l going from 1 to 61. When you compare the 4 different values for R they are rather similar. The same for the beta values.

Figure 7 shows the combined R results for 2 WMAP and 4 Planck simulations for l = 1 to 20. The 2 WMAP simulations are for the Hours 10 and 45. The 4 Planck simulations are for the Hours 10, 45 and 90 Each column with R values is a combination of 4 different simulations. For example column 3 "WMAP 45" shows the combined results of the data in Picture 10 and 11 When you compare the results of both WMAP and Planck for Hour = 10 than they are almost identical The same for the WMAP and Planck results for Hour = 45. But when you compare the results for Hour = 10 and Hour = 45 than they are different. This is more or less as expected because the WMAP and Planck results are generally speaking identical, but if you compare the results for different meridians than they are different.

l WMAP 10 Planck 10 WMAP 45 Planck 45 Planck 45* Planck 90 1 33,59 44,06 9,32 11,27 12,63 30,56 2 24,14 42,97 24,36 46,48 36,54 4,93 3 6,62 5,06 8,96 9,16 5,18 11,04 4 23,26 19,65 9,11 10,11 4,31 12,31 5 6,71 3,20 26,90 27,36 24,14 18,59 6 21,00 22,59 6,57 4,60 6,14 25,91 7 9,94 10,66 5,59 6,72 2,15 16,66 8 9,98 14,89 19,01 17,37 13,15 10,41 9 22,29 30,17 4,72 16,91 10,17 16,03 10 1,20 8,08 15,77 16,80 11,96 10,63 11 3,79 12,39 18,59 14,29 14,35 2,10 12 4,87 7,56 3,44 1,26 4,42 7,38 13 28,36 36,73 2,13 9,16 5,50 13,59 14 12,13 25,56 14,32 18,62 10,85 7,81 15 16,08 22,69 3,44 47,00 2,23 22,40 16 4,85 7,75 4,04 3,45 6,46 12,98 17 0,46 2,56 5,95 13,08 12,88 14,10 18 15,44 19,98 6,48 5,14 4,89 18,19 19 1,11 8,85 19,40 13,59 17,88 18,59 20 3,39 2,96 9,86 13,22 10,68 7,56 R values Figure 7

WMAP 10 38,07 Planck 10 94,28 WMAP 45 41,35 Planck 45 111,62 Planck 45* 91,51 Planck 90 98,91 Average error values Figure 8

Figure 8 Show the average error values for 2 WMAP simulations and 4 Planck simulations for 3 different Hour values. In most cases l goes from 1 to 60. In Planck 45* l goes from 1 to 500. The reason that the average error for the Planck results is higher (compared with the WMAP results) comes because the positiv temperature values are higher and the negatif values are lower. Specific the peaks are higher and the valleys are lower. The Planck 45* value is lower than the Planck 45 value because much more sinus functions are included (500 compared to 60)

The power spectrum of the whole sky shows an almost continuous curve for l going from 1 to 1500 with a clear peak at roughly l = 500.
Such a peak is not observed in the simulated sinus functions for each meridian.

When you study the results the question arises: What is the physical interpretation of each of these sinus functions.
IMO none. They have no physical meaning. They are not real. They do not describe a physical effect. It is only pure mathematics.
To claim that the sinus functions are Baryonic Acoustic Oscillations is not obvious.

The only exception is when you modify the function slightly and R(0) is included.
For the results see: Comparison with R(0)
For the WMAP results there is almost no difference
For the Planck results there is a difference because R(0) is negative. The CMB radiation images are fluctuations around an average temperature of 2.725 Kelvin. A negative value of R(0) means that this average value should be lower.

You could also perform the same exercise around earth as a function of the distance above and below sea level and calculate the same harmonic waves. Also for these harmonic waves there is no physical interpretation.

Doppler Boosting

One aspect which can be used to explain the above mentioned sinus functions is Doppler Boosting.
For a description of this phenomena read this: Planck 2013 results. XXVII. Doppler boosting of the CMB: Eppur si muove
IMO Doppler boosting can only be used for l = 1, assuming it is not taken into account. That means only for the function: R(1) * sin( alpha * pi/180 + beta(1))

Reflection

The most striking result of the Planck data is that there are many more hot and cold spots compared with the 9 year WMAP data.
See For example 7 Year WMAP probe observations: Cosmological Interpretation
The two most important question to answer are:

• First is what does the colour of each pixel represents and
• secondly what is the physical interpretation.

The colour of each pixel represents the wave length (or frequency) of the (CMB) radiation received. The colour red means: high temperature and high wave length (relative). Blue means low temperature and low wave length
However this raises a problem when you compare WMAP with Planck data.
When you consider the left corner of picture 3 you will see that this is an area with high temperatures. When you consider the same corner of picture 4 then you will see that this is also an area with high temperatures but the values are much higher.
That means that each pixel receives a range of photons with different wave lengths.The conclusion is that the final colour of each pixel represents an average of the received wave lengths.
The same is true for the low temperatures. For example the bottom right corner of Picture 3 and Picture 4.
The reality is that from each position in the sky we receive CMB radiation within a range of frequencies.

This fact makes the physical interpretation much more difficult. The frequencies outside the average value should also be included and they can be important to calculate the cosmological parameters. In fact they make the calculation more difficult.
This is different when you consider star light. For star light the main parameter is intensity. That means all photons of all frequencies are considered. You can also study the temperature of a star. In that case only a subset of all the frequencies are studied.

Reflection part 2

The same exercise as described above is also explained in the next document. CMB Power Animations
Specific in the first simulation which shows a magenta band which passes from left through right through the power spectrum. At the same time the simulation shows the corresponding hot and cold spots of the colour map. However these changes in the hot and cold spots are completely different compared with above.

The simulation goes from left to right, from large angles to small angles, from low accuracy to high accuracy, from COBE to WMAP to Planck data. However this simulation is not correct.

• The high accuracy data (right) shows tidy fluctuations but the overall colour is almost flat implying only a small temperature range. Planck data (picture 5) shows also tidy fluctuations but many colours implying a large temperature range.
• The low accuracy data (left) shows large fluctuations with two distinct colours, implying a large temperature range. Cobe data (picture 7) shows also large fluctuations but with the colours are less distinct, implying a much smaller temperature range.

For more about the CMB radiation read this: Friedmann's equation - Question 13
Back to my home page Contents of This Document